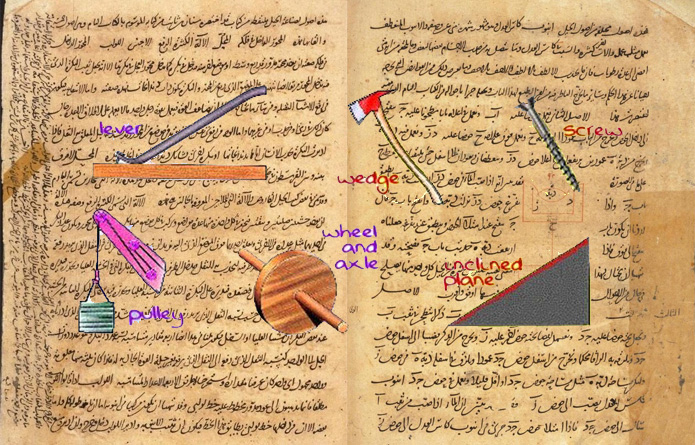
The Arabic manuscript Orient fol. 3306 preserved at the Staatsbibliothek in Berlin was in its original form a precious collection of Arabic scientific texts of mechanics and optics. It contains a fragment in one folio page consisting in a brief characterisation of the five simple machines: lever, windlass, pulley, wedge, and screw. This short text and is attributed to Sinān ibn Thābit, the son of Thābit ibn Qurra and a known mathematician and physician in Baghdad during the 10th century. It is a new source that has never been studied before. In the following article, we present the Arabic text of Sinan ibn Thabit and its English translation, accompanied with historical and analytical commentaries.

by Mohammed Abattouy[1]
***
Note of the editor
This article was first published as Mohammed Abattouy, “A New Arabic text of Mechanics: Sinan ibn Thabit on the Theory of Simple Machines”, in Studies on the History of Sciences, edited by Ja’far Aghayani Chavoshi, Tehran, 1390/2011, pp. 19-38.
***
Table of contents
***
The Arabic manuscript Orient fol. 3306 preserved at the Staatsbibliothek in Berlin[2] was in its original form a precious collection of Arabic scientific texts of mechanics, containing also two tracts on optics. The original content of the manuscript is given on the first page of the codex. Among the texts listed on this page we find the following title: Multaqatāt Kitāb al-tām li-Sinān b. Thābit fī dhikr ’uṣūl al-khamsa (sic) (Extracts from the Complete Book by Sinān b. Thābit in the mention of the principles of the five [simple machines]). This title corresponds to a fragment in one folio page in the codex, namely the folio 132r-v (fig. 1a-b). The recto page of the folio consists in a brief characterisation of the five simple machines, the “five powers” of the Ancients (lever, windlass, pulley, wedge, and screw), while we find on the verso the description of various other machines. It appears that these two fragments were extracted from a longer text entitled originally Al-kitāb al-tām (the Complete Book) by Sinān ibn Thābit. Obviously, this scholar is the son of the well known scientist Thābit ibn Qurra, and he was himself a mathematician and physician in Baghdad during the 10th century.
The fragment attributed to Sinān ibn Thābit on simple machines conserved in the MS 3306 is a completely new source that has never been studied before. In the following, the Arabic text and its English translation are presented, with historical and analytical commentaries, including a bio-bibliography of Sinān ibn Thābit.


Fig. 1 (a-b): The title of Sinān’s text on the first page of MS 3306 and the first line of the text on the top of folio 132r.
1. Description of MS 3306 and its contents
As it is attested by a note written on its first folio, the materials conserved in the MS Orient fol. 3306 of the Berliner Staatsbibliothek (State Library of Berlin) were copied or bound in one volume in 1090 H (1679). These materials form a collection of texts of physics (mainly mechanics and optics) of which the list is given on the first page of the codex. This collection contains 10 titles in the following order.
Among these titles, only two are conserved in the volume as it is preserved at present in Berlin’s Staatsbibliothek: a complete copy of the text of Al-Jazarī on machines in 132 folios, accompanied with beautiful drawings in colour, marked by a good artistic quality, and two fragments from the text attributed to Sinān ibn Thābit. These two fragments are written on the folio 132 recto-verso. This folio is inserted at the end of Al-Jazarī’s treatise. Obviously, this folio 132 is part of a longer text on mechanics, but unfortunately this is the only surviving part. That the folio was originally part of a longer text is indicated by the fluent and normal course of discourse at the end of folio 132v and the way the last word (’imtalā’) is written, below the last line, so as to provide a reference to the first word on the next folio, which is no longer extant in the codex.[4]
The two faces of folio 132 include the following materials. The recto displays a theory of simple machines that will be dealt with in the rest of this article. The verso contains descriptions of several mechanical devices (fig. 2a-b). The latter are referred to by a sentence which looks like a header (it is written in bold): “hādhihi uṣūl mukhtalifa min uṣūl al-ḥiyal” (these are various basic machines). Then begins the description of a machine: unbūb ka’s al-‛adl (the pipe of the cup of justice). At the end of the description, the author states that this device was described in full in “Al-Kitāb al-tām alladhī minhu ikhtaṣartu hādhā” (the Complete Book from which I summarized this [extract]).[5]




Fig. 2(a-b): Folio 132 recto and folio 132 verso. The first containing the text of Multaqaṭāt Kitāb al-tām li-Sinān b. Thābit fī dhikr uṣūl al-khamsa, whilst the second includes the description of various machines (’uṣūl al-ḥiyal), being part of Sinān ibn Thābit’s original treatise.
On the basis of the available data, it is possible to reconstruct the genesis of this text along three stages:
(1) First, Sinān ibn Thābit composed a book known as Al-Kitāb al-tām, of which at least a part is devoted to mechanical issues. Given the title of the book, we can suppose that the treatise has an encyclopaedic scope, and hence was conceived as a treatise on mathematical sciences in which a part deals with mechanics, including the theory of simple machines and the description of a series of simple devices.
(2) At least the section of Al-Kitāb al-tām devoted to the theory of simple machines was summarized (ikhtiṣār), probably by the author, namely Sinān ibn Thābit, but it is possible that this summary was achieved by another scholar.
(3) The fragment that survived on folio 132 in MS Berlin 3006 in its present form is an extract (multaqaṭāt) from this summary; as it was said above, such an extract may have been composed by another scholar than the author of the book. Several indications attest that the two pages of folio 132 are part of a longer text. The exceptional status of this folio 132 is that it represents the only surviving evidence known so far of Sinān’s Al-Kitāb al-tām and the unique source we know of mentioning his interest for mechanics.
The last folio of MS Berlin 3306, namely folio 133, includes the last two pages of al-Jazarī’s treatise. On the recto, we find a part of Al-Jazarī’s text about a machine “zawraq fīhi malāḥ wa-fīhi zammāra” (a boat with a sailor and a flute). Then begins the colophon of Al-Jazarī’s treatise preceded by the list of letters in abjad system used in the treatise (21 letters of the Arabic alphabet) and the symbols to which they correspond in the book. This means that originally the copy of Al-Jazarī’s work preserved in MS 3306 was complete.[6]
In the list of the contents of MS Berlin 3306 as well as in the title of the text on simple machines, the fragment in folio 132 is attributed to Sinān ibn Thābit. That the author of this text is Sinān ibn Thābit, the son of Thābit ibn Qurra, is supported by different arguments. First of all, the text is ascribed in the manuscript to Sinān ibn Thābit, identified as the son of Thābit ibn Qurra by the editors of the German catalogue where MS 3306 is mentioned.[7] Furthermore, several indications attest that the fragment is of an early date and may have been written by Sinān in the first half of the 10th century. This is confirmed by the archaic style of the vocabulary, as it is shown by the following two significant instances: the lever is called muḥl; later on, the standard word for it was bārim or bayram; the same for qarasṭūn (= steelyard), transformed later on as qaffān and qabbān.[8]
On the other hand, as far as we know, there exists no scholar in the Islamic scholarship bearing the name Sinān ibn Thābit, but Abū Sa‛īd, the son of Thābit ibn Qurra. In addition, the text is bound with several early Arabic writings that still reflect traces of the Graeco-Arabic transmission period, as it is clearly shown in the table of contents of the codex. Nevertheless, no historical source ever mentioned a writing of Sinān ibn Thābit in mechanics, although this should not be considered a priori as a counter-argument to deny the possible existence of such an interest. The ongoing investigation on the corpus of Arabic mechanics and engineering and its achievements is changing; new discoveries were made and others are still to come. This has been proved in the field of theoretical mechanics, with the dramatic change generated by the recent research on the science of weight (‛ilm al-athqāl).[9]
The MS Berlin 3306 was published online on the website of the Max Planck Institute for the History of Science in Berlin, as a result of the investigation performed by the author of this article on this document in the Staatsbibliothek in Berlin. This electronic edition may be accessed here: Anonymus, Ms. or. fol. 3306, Arabisches manuscript.
2. Bio-bibliography of Sinān ibn Thābit
Abū Sa‛īd Sinān ibn Thābit ibn Qurra al-Ḥarrānī is a well known scholar of the 10th century. He is mentioned in the classical and modern sources as a mathematician, astronomer, physician and historian. He was the son of Thābit ibn Qurra (d. 288 H/ 901), the famous Harranian scholar who flourished in Baghdad and excelled in different fields of science and medicine, including mathematics, astronomy and mechanics. Two sons of Abū Sa‛īd Sinān ibn Thābit distinguished themselves in science and medicine. The first one, Abū Isḥāq Ibrāhīm ibn Sinān, was a genius mathematician. He left valuable works, such as his famous treatise on analysis and synthesis. He died in Baghdad in 335 H/946-947 when he was only 38 years old.[10] The second one is Abū ‘l-Ḥasan Thābit b. Sinān (b. Thābit b. Qurra) who excelled in medicine and died in 363 H/973-73.[11]
Sinān ibn Thābit was the personal physician of two Abbasid Caliphs, Al-Muqtadir (r. 908-932) and Al-Qāhir (r. 932-934). He served also Al-Rādhī (934-940), with whom he entertained good relationships. The latter asked him to convert to Islam, but Sinān was not ready to embrace Islam and change his religion. Being afraid to disobey the Caliph’s request, he fled to Khurasan. He came back to Baghdad after the destitution of Al-Qāhir. But later on, he converted to Islam and died in Baghdad as a Muslim at the beginning of Dhū-‘l-qi‛da 331 H (August 943).[12]
Sinān ibn Thābit was the representative of the second generation of Harranian scientists and physicians in the Abbasid court in Baghdad, in the aftermath of the arrival and settlement of his father Thābit ibn Qurra in the Abbasids’ capital in the middle of the 9th century. By converting to Islam, he put an end to the Sabian tradition of the prestigious family his father had founded in the capital of the Muslim empire, and strengthened its integration in the Muslim society. For a long time before and after him, scholars originating from Harran occupied eminent positions among the intellectual, scientific and medical elite at the caliphal court and in the high spheres of society.
Ibn abī ’Uṣaybi‛a mentioned that Abū Sa‛īd Sinān ibn Thābit was well versed in the sciences like his father, and he was gifted in astronomy and in the art of medicine. He described in detail the efforts deployed by Sinān ibn Thābit in the organisation of hospitals and enumerated the moral qualities of Sinān, such as his commitment under Al-Muqtadir to provide medical care to prisoners in Baghdad and to poor people in the suburbs of the city.[13]
Besides his distinction in medicine and astronomy, Sinān was also a historian and a gifted mathematician. Ibn al-Nadīm ascribed to him two texts in mathematics: Kitāb fī ‘l-istiwā‛ (Book of levelling), and Iṣlāḥuhu li-kitāb (…)[14] fī al-‛uṣul al-handasiyya, wa zāda fī hādhā al-kitāb shay‛an kathīran (his revision/edition of The Book… of Geometrical Principles, to which he added a great deal). Later on, Ibn al-Qiftī provides the full title of this book and states that this treatise of ’uṣūl handasiyya that Sinān ibn Thābit edited and augmented was the work of A/Iflāṭun (Plato?).[15] It is possible to conjecture that this book of ‘uṣūl‘ worked out by Sinān ibn Thābit was a book of mathematical sciences in which our scholar enriched a Greek original text, and added one or more chapter, one of them dealing with mechanics. In this case, this book should bear in certain copies the name of Al-Kitāb al-tām, from which the fragment of ‘’uṣūl‘ on simple machines was extracted. However, no evidence is available to support such a conjecture, and one is bound to suppose that al-Kitāb al-tām stands as an independent writing of Sinān, even though none of his bio-bibliographers made any mention of it.
Other works of mathematics are attributed to Sinān ibn Thābit, such as his edition of a text of Archimedes on the triangles, translated previously by Yūsuf al-Qiss from Syriac.[16] The bio-bibliographers mention also that he performed the revision of the works of the mathematician al-Kūhī (Iṣlāḥuhu li-‛ibārat Abī Sahl al-Kūhī fī jamī‛i kutubihi). The fact that al-Kūhī asked Sinān to revise his writings is a sign of competence and recognition of his abilities, and testifies to the privileged relationships between the two scholars. Given what we know of Sinān’s work, we can suppose that the revision went far beyond the linguistic and stylistic presentation of the texts to reach certain aspects of the contents. [17]
3. Survey of the Arabic tradition of the five simple machines
As far as we know, no historical source mentioned that Sinān ibn Thābit wrote a text of mechanics titled Al-Kitāb al-tām nor reported about his interest in mechanics. Thus, the material preserved in Codex Berlin 3306 and ascribed to this author is a new and unknown component of the Arabic corpus of mechanics, and one of the rare Arabic writings on the theory of simple machines or the five powers. This theory was directly inspired from Greek works translated into Arabic, such as the mechanical treatises of Pseudo-Aristotle, Heron and Pappus. Besides Sinān ibn Thābit’s fragment, the main treatises which we know of today as having developed the theory of the five simple machines in Arabic mechanics are: Mi‛yār al-‛uqūl, a Persian treatise attributed to Ibn Sīnā and Al-Isfizārī’s summary of the second book of Heron’s Mechanics.
Mi‛yār al-‛uqūl dur fan jar athqāl (The Measure of mind or the art of dragging weights) is a Persian treatise attributed to Abū ‛Alī al-Ḥusayn ibn Sīnā (980-1037). It deals with the description of the five simple machines and of their use in displacing heavy loads. As such, the text is a precious source for the reconstruction of the theory of the five powers in Islamic science in the 10th and 11th centuries. Mi‛yār al-‛uqūl is not just a mere summary of the Greek theory of simple machines as it was transmitted in Hellenistic sources. It represents the first systematic classification of those machines, individually and in combination.[18]
Mi‛yār al-‛uqūl describes the five simple machines in four chapters. In the first two chapters, the author follows closely Heron’s characterization of the five powers, and borrows the essential part of his descriptions and drawings of the simple machines from Heron’s Mechanics. The machines are quoted in this order: miḥwar (windlass), muḥl (lever), bakara (pulley, wheel), lawlab (screw) and isfīn (wedge).[19] The description of each machine is accompanied by a diagram and illustrated by a geometrical figure. The second part of the treatise, composed of chapters 3 and 4, contains descriptions of combinations of the five simple machines. Like Heron, ibn Sīnā classifies these combinations by the principle of likeness or unlikeness of the constituent five powers. Thus, in chapter 3, he describes combinations of like simple machines: windlasses, pulleys and levers. Then, in chapter 4, he goes beyond the limits of Heron’s classification when he successively analyses all probable combinations and considers all practically probable pair wise combinations of unlike simple machines: windlass-pulley, windlass-lever, windlass-screw. Finally, in chapter 4-section 5, he describes a mechanism which is essentially a combination of four simple machines; only the wedge is left apart (fig. 3a-b).


Fig. 3 (a-b): The five simple machines of the ancients, plus the “inclined plane”, a device to which modern mechanicians reduced the wedge and the screw. Adapted from Unit 3: Simple machines, p. 2.
Another work in the Islamic tradition on simple machines is contained in Al-Isfizārī’s epitome of the second book of Heron’s Mechanics. This summarized version exists in two known manuscript sources: MS 351 at the John Rylands Library in Manchester (UK) and MS Q‛620 H-G at the ‛Uthmāniyya University Library in Hyderabad (India). Composed of a series of epitomes and commentaries on selected parts of the mechanical works of Heron, Apollonius and Banū Mūsā, this text has never been studied nor edited and was mentioned so far only in some studies by the author of this article (fig. 4).[20]


Fig. 4 (a-b): The five simple machines in the Arabic version of Heron’s Mechanics: (1) the windlass, (2) the lever, (3) the wedge, (4) the pulley and (5) the screw. Source: Andhra Pradesh Government Oriental Manuscripts Library and Research Institute in Hyderabad, MS Riyādhī 396, respectively on folios 14v, 15v, and 16r.
4. The Arabic text and English translation
In the following, a transcription of the text of Sinān’s fragment is provided, with English translation. It should be pointed out that because of difficulties in reading some words of the last paragraph in the manuscript; the transcription proposed hereinafter for the last lines of the text is tentative only, until we have access to this fragment in another manuscript source.
On the other hand, as it may be seen on figure 5, the last part of the text is written in four lines on the left hand margin of the page. This may be due to the desire of the copyist to include all the text of Sinān’s treatment of the theory of simple machines on the same page (perhaps for better reading).


Fig. 5: Facsimile of the folio page 132r of Sinān ibn Thābit’s fragment on simple machines in MS 3306. Source: The Staatsbibliothek in Berlin, electronic edition, Anonym., Ms. or. fol. 3306 – Arabisches manuscript.
Multaqatā [min al-]Kitāb al-tām li-Sinān ibn Thābit fī dhikr al-uṣūl al-khamsa
Extracts from the Complete Book by Sinān ibn Thābit on the Five Powers
| These are basic principles of the art of mechanics extracted from a book which has been summarized by Sinān ibn Thābit from his book called The Complete Book. |
هذه أصول لصناعة الحيل ملتقطة من كتاب قد اختصره سنان بن ثابت من كتابه الموسوم بالكتاب التام. |
| These are five basic [machines], and their names are: the axle introduced in a wheel [the windlass], the lever, the pulley, the wedge and the screw. |
وهي أصول خمسة وألقابها هذه: المحور الداخل في فلكة، المحل، الآلة الكثيرة الرفع، الأجنة،[21] اللولب. |
| The axle introduced in a wheel: Its description: you take a round axle of which the middle or any other part has been squared, and it is introduced in a wheel as the axle of the cart-wheel is introduced in its wheel. |
المحور الداخل في فلكة: صفته أن تتخذ محور مدور قد رُبِّع وسطه أو موضع آخر منه فيدخل في بكرة كما يدخل محور العجل في بكرتها الداخل في فلكة. |
| But the hole of the wheel in which the axle is introduced is made squared also, fitting on the squared part of the axle. The wheel must have teeth/cogs. This is its description. |
إلا أنه يجعل ثقب البكرة الذي فيه يدخل المحور مربعا أيضا متهندم على الموضع الذي ربع من المحور والبكرة تكون ذات دندانجات، فهذه صفته. |
| As for the utility drawn from it, it is [used] for raising heavy things and in all what requires the multiplication of force, so that the force of one single man may do what can be done definitely by several men only, and this either in throwing or in pulling or otherwise. |
وأما الإنتفاع به فيكون في رفع الأشياء الثقيلة وفي سائر ما يحتاج فيه إلى أن تضاعف القوة، فيعمل بقوة رجل واحد ما لا يعمله على الإطلاق إلا عدة رجال، كان ذلك في رمي أو في جذب أو في غيرهما. |
| The method to raise a heavy thing with it is that a spoke is spoked in a place of the axle and to which is fastened the rope by which the weight is suspended. When the wheel revolves, it is pulled by its cogs or by another wheel with the cogs of which its cogs are entangled. |
وأما الوجه في رفع شيء ثقيل به بأن يوتـّـد في موضع من المحور وتد يشدّ به الحبل المعلق به الثقل، فإذا أديرت البكرة تجذب الأسنان إياها بدندانجاتها أو ببكرة أخرى تشابك دندانجاتها دندانجات هذه. |
| The lever: There is no difference between it and the steelyard (qarastūn) in its theory. It is the principle to which most of the matter of heaviness and lightness is reduced. |
المحل: لا فرق بينه وبين القرسطون في حكمه، وهو الأصل الذي إليه يرجع أكثر أمر الثقل والخفة.
|
| The lever is this instrument used by the carpenters which is called al-bārim. It is called in some books of mechanics al-qārūs. The instrument used by sailors known as al-jālis is this one. |
والمحل هو هذه الآلة التي للنجارين تسمى البارم، وقد سمي في بعض كتب الحيل القاروص. والآلة التي للملاحين المعروفة بالجالس[22] هي هذه. |
| [The beginning of the paragraph on the pulley is missing]
The description of this instrument is that we take two hard and solid wooden plates. We dig in each one of them at several places and we set at each place a wheel which turns therein on an axle. The number of wheels in one of them should equal those in the other. We suspend one of the plates above the position to which we want to raise the weight and [we attach] the other to the weight which we want to raise. |
[…] وصفة هذه الآلة أن تؤخذ خشبتين صلبتين وثيقتين فنحفر في كل واحدة منها عدة مواضع ونركب في كل موضع منها بكرة تدور فيه على محور. ويكون عدد البكر في أحديها مثل عدده في الأخرى. ونعلق أحديهما فوق الموضع الذي إليه نريد أن نرفع الثقل، والأخرى في الثقل الذي نريد رفعه. |
| Then we take a long rope and we grasp one of its ends so that the weight may be pulled by it. We introduce the other end in one of the wheels attached to the higher plate. We lower it and revolve it around one of the wheels attached to the lower plate. We lift it and revolve it on the second wheel attached to the higher plate. We lower it and revolve it on the second wheel attached to the lower plate. |
ونأخذ حبلا طويلا فيمسك أحد طرفيه ليجذب به الثقل فيدخل الطرف الآخر في إحدى البكر التي في الخشبة العليا، ونحدره فنديره على بكرة من التي في الخشبة السفلى، ونصعده فنديره على البكرة الثانية من الخشبة العليا ونحدره فنديره على البكرة الثانية من الخشبة السفلى. |
| We keep repeating this according to the ratio of the weight we want to raise to the pulling force. Then we fasten the end of the rope that we have revolved to one of the wheels, be it one of the highers or one of the lowers. We have then four proportional magnitudes: |
ولا نزال نكرر ذلك على حسب نسبة الثقل الذي نريد رفعه إلى القوة الجاذبة. فنشد حينئذ طرف الحبل الذي أدرناه عند بعض البكر، إن شئنا العليا أو السفلى، فتكون لنا في هذا أيضا أربعة مقادير متناسبة: |
| The ratio of the number of wheels, which is also the number of revolutions of the rope, to one is as the ratio of the raised weight to the weight raising it without the intermediary of the force pulling it. If three of them are known and one is unknown, whatever it is, we may know its magnitude and determine it by the method of the four proportional numbers. |
نسبة عدد البكر وهو عدد دورات الحبل إلى الواحد كنسبة الثقل الذي رفع إلى الثقل الذي يرفعه بغير حيلة القوة الجاذبة له. فإذا كان ثلاثة منها معلومة وواحد مجهول أي واحد كان عرفنا مقداره واستخرجناها بطريق الأربعة الأعداد المتناسبة. |
| The screw: If it is said absolutely, the screw means a rounded [piece of] wood on which a screwed line (khaṭ lawlabī) is marked. It may be composed of a pipe which occupies its length in whole or in part. |
اللولب: إذا قالوا اللولب مطلقا فإنما يذهبون إلى عود مدور مخطوط عليه خط لولبي وقد يتهيأ أن يكون مركبا من أنبوبة إما أن تأخذ طوله كله وإما بعضه. |
| But it has inside a screwed line corresponding to this line so that when the pipe is kept fixed while the screw is revolved, it goes in and out. If it is revolved and prevented from going in and out, it enters in the pipe…[23] With the condition that the screw is set on a plane circle so that the… [24] |
إلا أن في داخلها خط لولبي يطابق هذا الخط فيكون إن ثبتت الأنبوبة وأدير اللولب دخل وخرج. وإن أدير ومنع[25] من الخروج والدخول دخل إلى الأنبوبة وخرج،[26] هذا إن كان/وذلك أن يكون اللولب مركبا على دائرة مشرحة فيكون المدبب (؟) فيه. |
| It occurs in this instrument, I mean the screw, a motion composed of two motions, the circular motion and the straightforward motion. In the screw having closer lines (al-mutaqārib al-khuṭūṭ) the circular motion dominates (aghlab) and its rotation is easier. Hence the weight it raises is greater and the time of its lifting is longer. |
وهذه الآلة أعني اللولب تعرض منها حركة مؤلفة من حركتين حركة الإستدارة وحركة الإستقامة. واللولب المتقارب الخطوط حركة الإستدارة فيه أغلب وإدارته أسهل فالثقل الذي يرفعه أعظم وزمان إرتفاعه أطول. |
| In the screw having wider lines the motion of straightness dominates, and it acts contrarily to the one having closer lines, with respect to the magnitude of what it raises, to the time in which it raises it and in every other circumstance. It is by these two motions that we mark the screwed line. |
واللولب المتباعد الخطوط فالإستقامة في حركته أغلب وهو في مقدار ما يرفع وفي الزمان الذي يرفعه فيه وفي سائر الأحوال على ضد ما عليه المتقارب الخطوط وبهاتين الحركتين نخط الخط اللولبي. |
| The wedge |
الأجنة[27] [الإسفين] |
| Its utility is in the matter of mechanics (’amr al-ḥiyal) and it is very rarely used [outside of it]. It acts in one exclusive place where it does a wonderful action can not be replaced[28] by anything else amongst the rest of the five powers (al-khams uṣul)[29], namely in cutting and extracting stones (qatc… qalc), and in sum in splitting (shaq) everything that may be splitted…[30] |
فالإنتفاع به في أمر الحيل وإستعماله قليل جدا وله موضع واحد يفعل فيه فعلا عجيبا ولا مناص منه فيه ولا ينوب عنه شيء من باقي الخمس أصول، وهو قطع الحجارة وقلعها، وبالجملة شق كل شيء يشق. وكانوا ربما إستعملوه في سور المدينة أو في الحفر ومايشابهه…[31] |
5. Short analysis of the contents of the text
Sinān ibn Thābit’s text presents a summarized theory of the five simple machines in the form of short descriptions of their functions. The machines are identified on the basis of two procedures: terminological meaning of their names and practical considerations on their use.
The five simple machines dealt with are: the windlass, the lever, the pulley, the screw and the wedge. These machines are called by the same Arabic terms employed in the translation of the Greek names of these instruments. The only difference is the name given to the wedge. Instead of the usual Arabic term used for this instrument, which is al-isfīn, it is called in MS 3306 as al-ajanan, probably an Arabization of its name in Greek, Syriac or Persian (fig. 6).


The order in which the simple machines are quoted in the major sources that discussed the theory of simple machines in Greek and Arabic mechanics seem to be Book II of Heron’s Mechanics. The only exception is the Mi‛yār al-‛uqūl ascribed to Ibn Sīnā, where the wedge is dealt with before the screw. For comparison, the simple machines are quoted in Pseudo-Aristotle’s Mechanical Problems in the following relative order: the lever, the windlass, the wedge and the pulley, the lever being considered the basic machine to which all other simple machines are to be reduced, especially the windlass and the pulley.
The fact that the lever is not quoted in the first place in Sinān’s text may testify an inclination to discard the Peripatetic view which consists in the reduction of all the simple machines to the lever, and thus to the circular motion it represents. On the other hand, Sinān ibn Thābit’s text establishes a clear connection between the lever and the steelyard, referred to as qarasṭūn, the name of Greek origin that prevailed for this instrument in Arabic scientific literature in the 9th and 10th century. Thābit ibn Qurra, the father of Sinān, wrote a very influential treatise on it under the title Kitāb fī ‘l-qarasṭūn.
The early date of Sinān’s text is attested by his vocabulary, which is reminiscent of the Arabic technical terminology derived from the translation of the Greek sources of mechanics. Hence, the steelyard is called qarasṭūn and not qabbān or qaffān (the last two terms are found in texts dating from the second half of the 11th century). Further, the lever is designated by muḥl and bārim. This synonymy denotes a transition between muḥl, directly derived from the Greek mochlos, and al-bayram, the standard Arabic word (of Persian origin) used by al-Khāzinī in Kitāb mīzān al-ḥikma (12th century). On the other hand, Al-Khwārizmī’s 10th-century text Mafātīḥ al-‛ulūm establishes an explicit synonymy between the two when it identifies mukhl (muḥl) and bayram–bārim. In the same section, Sinān affords two other Arabic names for the lever: qāruṣ and jālis. As far as we know, these two terms are associated to the lever only in his text. In addition, the mention of two early and exotic terms for the lever (qārūs and jālis) denotes an early origin of the text, since these terms are not mentioned in any other later Arabic mechanical text.
Sinān’s description of the machines is similar to Heron’s nomenclature of these basic instruments, but with some refinements. This is the case of his description of the windlass. The characterization of the wedge as the only simple machine that cannot be replaced by any other one of the four other simple machines is identical in Heron and in Sinān. But the latter’s description of the lever is an admirable concentrated piece of theory. It establishes a direct analogy between this simple instrument and the steelyard. This analogy is reminiscent of a similar approach in Pseudo-Aristotle’s Mechanical Problems, even though Sinān’s remark that most of the problems of heaviness and lightness are to be referred to the properties of the lever goes beyond the limits of the analogy established in Pseudo-Aristotle between the lever and the balance. The son of Thābit ibn Qurra was more likely referring here to a well-established Arabic tradition of study of the steelyard in his time that made this instrument the model of the balance and of the other simple machines.
It is worthwhile to note that Sinān talks of the five simple machines in terms of ’aṣl (pl. ’uṣūl), meaning root, origin, and basic principle. In this perspective, Al-’usūl al-khams refer to the basic fundamental machines, on the basis of which other instruments may be conceived (they would be furū‛, consequences derived from them). This terminology is rooted in a usual dichotomy in Arabic thought, opposing ’uṣūl to furū‛, basic and primary things to consequences derived from them. In this context, the five powers are the basic machines from the combination of which other machines may be derived. Now the classification of machines and their combination is an important theme of Arabic mechanics, as for example in Mi‛yār al-‛uqūl attributed to Ibn Sīnā. It is thus possible that later sections in the complete version of Sinān ibn Thābit’s work were precisely devoted to the classification of machines and their combination.
Finally, two interesting terminological instances are to be specially noted in Sinān’s text. First, he used in the section about the pulley the word ḥīla in the sense of means, intermediary. This is exactly one of the original senses of mechane in Greek. Secondly, the idea of the composition of motion in the section on the screw is worth to be highlighted also. The working of this machine is said to be generated by two motions, a circular one and a straight one (ḥaraka mu’allafa min ḥarakatayn, ḥarakat al-istidāra wa-ḥarakat al-istiqāma). There is no such clear and explicit idea of the composition of motions in the screw in Heron’s discussion of this machine in the second book of his Mechanics.
6. Appendix: Description of the contents of MS 3306
The list of the texts mentioned on the first page of MS 3306 contains ten items. It is presented in the following in three forms: facsimile reproduction, transcription (both in fig. 7a-b), and in English translation, with commentaries on the titles of the texts.


Fig. 7 (a-b): The first page of MS Berlin Staatsbibliothek 3306 and its transcription in Arabic.
English translation
“The books collected in this volume are:
Commentaries on the titles
1. (Risālat al-Jazarī fī a‛māl al-ḥiyal (Treatise of al-Jazarī on the construction/making of machines): this is a complete copy of Al-Jāmi‛ bayna al-‛ilm wa al-‛amal al-nāfi‛ fī ṣinā‛at al-ḥiyal, the well-known work of Al-Jazarī (completed in 1206). This manuscript copy was not used in the recent edition and translation of al-Jazarī’s treatise.[32] It occupies ff. 1b-131b and f. 133a-b. The short text of one folio of Sinān ibn Thābit is intercalated at its end on f. 132r-b.
2. Multaqatāt Kitāb al-tām (sic) li-Sinān b. Thābit fī dhikr uṣūl al-khamsa (sic) (Extracts from The Complete Book of Sinān ibn Thābit on the Five Powers): This short fragment is the subject matter of the present article.
3. Maqālat al-Khāzinī[33] fī ‛amal kura tadūr bi-dhātiha (Treatise of Al-Khāzinī on the construction of a sphere that rotates by itself): Abū ‘l-Fath ‛Abd al-Raḥmān al-Khāzinī flourished in Khurāsān around 1115- 31. The main domains of his scientific activity are astronomy, mechanics and scientific instruments. He is the author of the famous Kitāb mīzān al-ḥikma, the book of the balance of wisdom. His other known works include a treatise on astronomical instruments (Risāla fī ‘l-ālāt) and Maqāla fī itikhādh kura tadūr bi-dhātihā, the text on the sphere that rotates by itself mentioned in this entry in MS Berlin 3306 (fig. 8).


Fig. 8 : The drawing of Al-Khāzinī’s sphere. Source: Maqāla fī itikhādh kura tadūr bi-dhātihā, The Syrian National Library in Damascus, Al-Ẓahiriya Collection, MS 4871, folio 73r.
3. This text is known at present in two manuscript copies preserved respectively at the Syrian National Library in Damascus (al-Ẓahiriya Collection, MS 4871, ff. 73r-74r), and in the oriental collection at Oxford Library (MS Thurston 3, ff. 118-119r). It describes a celestial globe worked with weights. The instrument has the form of a solid sphere marked with the stars and the standard celestial circles and half sunk in a box. Its rotation is propelled by a weight falling in a leaking reservoir of sand. The sphere is mounted so as to rotate once a day. It functions like an automatic celestial instrument, and may be used to find directly several arcs of importance in spherical astronomy. [34]
4. Sharḥ kitāb Biyanius[35] (?) al-ḥakīm fī ṣan‛at marāyā (sic) al-muḥriqa (Commentary on the book of Biyanius (?) the wise on the construction of the burning mirrors): The name of the author – obviously a Greek scholar – is without diacritic marks. The text belongs to an established tradition of works translated from the Greek or composed in Arabic on burning mirrors.[36]
5. Risāla fī … li-ma‛rifat al-sā‛a wa anwā‛ al-rukhāma wa-ghayruhu (Treatise on … for the determination of the hour and the kinds of the rukhāma and other things): The text is on an astronomical instrument, a sort of gnomon for measuring time. Thābit ibn Qurra has written a text with a similar title: Kitāb fī ālāt al-sā‛āt allatī tusammā rukhāmāt (Book on the instruments which give the hours, called the solar quadrants (rukhāmāt).[37]
6. (Kitāb Uqlīdīs al-ḥakim fī ‛ilm al-manāẓir wa kayfiyyāt al-shu‛ā‛ (The Book of Euclid on the science of optics and the qualities of the ray). This text stems obviously from the tradition of the Arabic edition of Euclid’s Optics, but it bears a title completely different from all those occurring in the Arabic tradition. The title quoted in MS 3306 might be a very early instance of this Arabic tradition of Pseudo-Euclid’s Manāẓir. The reference it contains to the shu‛ā‛ (ray) constitutes a valuable reference to an important feature of this tradition, the Euclidean ray theory.[38]
7. Unreadable title.
8. (Risāla fī ‛amal anwa‛ al-dawālīb al-mudawwara min tilqā’ dhātihā (Treatise on the making of different wheels that turn by themselves): seems to deal with self-rotating wheels, a topic of Arabic mechanics to which several treatises are devoted. An important text of this category occurs in several manuscripts with the title: Bakarāt tadūr min dhātihā (or min tilqā’ dhātihā) (Wheels that move by themselves). It has usually been assumed that it is a discussion of perpetual motion and as such dismissed as of no practical significance. The descriptions are difficult to understand and the accompanying illustrations are obscure. Nevertheless, the machines appear to embody important mechanisms and they would certainly repay further detailed study.[39]
The affiliation between item 3 in MS 3306 and this text on perpetual motion can be established on the terminological basis: maḥāla and bakara are synonyms and they refer to the wheel. Furthermore, if this is established, this may give a clue to determine the author and the date of both works. The author of the former is indeed given as al-Khārijī, probably a deformation of the name of Muḥammad ibn al-Ḥusayn al-Karajī, a known mathematician of the 10th-11th century (d. ca. 1016). He is also author of a work on hidden waters (Kitāb inbāt al-miyyāh al-khafiyya).[40] This topic, connected with engineering and levelling, was considered traditionally as a branch of mechanics.
9.(Unreadable title).
10. Fawā’id fī ma‛rifat mīzān al-‛adl wa-ghayruhu: may be rendered as “Advantages/Utilities about the balance of justice”. It deals probably with a mechanical balance. The balance is considered basically in the Islamic tradition as an instrument of justice, as it is clearly stated by al-Khāzinī in his Kitāb mīzān al-ḥikma.[41]
[1] Professor of History and Philosophy of Science, Mohammed V University, Rabat, Morocco. Senior Research Fellow, Foundation for Science, Technology and Civilisation, Manchester, UK, and Chief Editor of MuslimHeritage.com.
[2] I thank the Department of Oriental Manuscripts at the Staatsbibliothek in Berlin for allowing me to consult the MS Orient fol. 3306 in September 1999 and for providing me with a paper copy of some folios of the Codex. Work on this article began in Berlin when I was a visiting scholar at the Max Planck Institute for the History of Science (1996-2003).
[3] Two items of the list of contents could not be deciphered: see fig. 9. The codex is described in the vol. 17 of the series Verzeichnis der orientalischen Handschriften in Deutschland (List of the oriental manuscripts in Germany): Voigt 1990, pp. 149-152. However, this description leaves unanswered the question of the provenience of the volume and the fate of the leaflets containing the nine texts originally included in it. On the first folio, we find the accession number, acc. Ms 1917.1, probably the date of its purchasing in a German library. For more details on the contents of the manuscript, see the Appendix below. Just under the list of the texts bound originally in the codex, the first page of MS Berlin 3306 provides some data on the ownership of the manuscript and a precious date: the text seems to have been copied in Rabī‛ al awwal 1090 H by a certain Ibn Muhammad… al-Sharīf. Unfortunately, the rest of the name is covered by a stamp, which makes it difficult to identify this person.
[4] In this respect, it is safe to suppose that the loss of the rest of the text to which the content on folio 132 belongs is due to accidental circumstances. Obviously, the items documented in the table of contents of MS Berlin 3306 were in full assembled in the codex; their loss may have happened before the manuscript was transferred to the German modern collections.
[5] The verso of folio 132 contains the description of two other machines refered to as Al-‘asl al-thānī and ‘Asl thālith (respectively the second and third machines).
[6] However, this manuscript copy was not used in the edition and translation of al-Jazarī’s treatise by Hill 1974 and al-Hassan 1979.
[7] See Voight 1990, p. 200.
[8] For supplementary clues going in the same direction, see below Section 5.
[9] For a general survey on the research carried out in the last years on the Arabic science of weights and its signification for the reconstruction of the different traditions of medieval mechanics, see Abattouy 2006 and Abattouy 2007b.
[10] See the biography and works of Ibrāhīm ibn Sinān in the following classical sources and modern scholarly works: Ibn al-Nadim 1871-72, vol. 1, p. 272, Sezgin 1974, pp. 292-295, Sezgin 1978, pp. 193-195, and John J. O’Connor, Edmund F. Robertson’s online article Ibrahim ibn Sinan ibn Thabit ibn Qurra, MacTutor History of Mathematics Archive. His works are edited and analysed in Sa‛īdān 1983, Rashed 1996 and Rashed & Belhosta 2000.
[11] Ibn al-Nadīm 1871-72, vol. 1, p. 302; and Ibn abī ’Uṣaybi‛a 1965, pp. 304-07.
[12] The story of Sinān’s conversion is narrated by Ibn al-Nadīm 1871-72, vol. 1, p. 302, Ibn Abī ’Uṣaybi‛a 1965, p. 300, and Ibn al-Qiftī 1903, p. 190.
[13] Ibn Abī ’Uṣaybi‛a 1965, pp. 301-304; Ibn al-Qiftī 1903, pp. 193-194.
[14] Blank in the manuscript.
[15] The editor of Ibn al-Qifṭī’s book notes here that the manuscript bears at this place “Aqātun”. On the basis of this information reported in the Arabic sources concerning the work of Sinān on a book of “geometrical principles”, Heath, in his commentary to the English translation of Euclid’s Elements, stated that Sinān b. Thābit “wrote an ‛improvement of the book of… on the Elements of Geometry, in which he made various additions to the original.’ It is natural to conjecture that Euclid is the name missing in this description… The latest editor of the Tārīkh al-ḥukamā’ [by Ibn al-Qifṭi], however, makes the name to be Iflaton (=Plato), and he refers to the statement by the Fihrist… attributing to Plato a work on the Elements of Geometry translated by Qusṭā ibn Lūqā. It is just possible, therefore, that at the time of Qustā [mid-9th century] the Arabs were acquainted with a book on the Elements of Geometry translated from the Greek, which they attributed to Plato”: see Euclide 1956, p. 88.
[16] Ibn al-Nadīm 1871-72, vol. 1, p. 302; Ibn abī ’Usaybi‛a 1965, p. 304; Ibn al-Qiftī 1903, p. 195; Sezgin 1974, p. 291.
[17] Sinān ibn Thābit’s reputation as a mathematician is confirmed also by the historian Al- Mas‛ūdī (d. 346 H) in Murūj al-dhahab: Al-Mas‛ūdī 1973, vol. 1, pp. 16-17.
[18] See the the recent edition of the original text (Ibn Sīnā [1952]) and the short chracterisation of its contents in Rozhanskaya 1996, pp. 633-634.
[19] This is also the order in which these machines were studied in Sinān’s text: see below, section 5.
[20] See Abattouy 2000. Al-Isfizari’s works of mechanics are being edited, translated into English and commented upon by Mohammed Abattouy and Salim Al-Hassani in the framework of a research project sponsored by Al-Furqan Islamic Heritage Foundation in London. The outcome of this project will appear soon in a book: see The Isfizari project.
[21] Ajanan in MS.
[22] This word corresponds to the latin calix, already used by the Roman engineer Sextus Julius Frontinus (ca. 40-103 CE) in the sense of ‛conduit of acqueduct’. This sense is approximate to the qāllus that occurs in the Arabic mechanical texts (for instance, in the translation of Heron’s and Pappus’ Mechanics) where it means a rope.
[23] A corruption in the page here prevents from reading the word.
[24] The last two words of the phrase are not readable.
[25] The rest of the text is written, in the same handwriting, in four lines occupying the left margin of the page on folio 132r (see fig. 5).
[26] Not clear in the manuscript.
[27] Ajana is used until today for the wedge; for example, in Egypt, where it means a sharp knife.
[28] A corruption in the page here prevents from reading several words; the proposed sentence tends to replace the approximate meaning, which is apparently that the role of the wedge “can not be replaced” by any other machine.
[29] In the MS, “khams ṣūl“, which is evidently an orthographic slip.
[30] The last 10 words are without clear meaning. They are about the use of the wedge in the “wall of the town” (sūr al-madīna).
[31] Several words of this phrase are not clear in the manuscript; the proposed reading is a tentative reconstruction.
[32] See Hill 1974 and al-Hasan 1979.
[33] Without diacritic points.
[34] This short description of al-Khazini’s device is extracted from Abattouy 2007a. A full description of the instrument is given in Emilie Savage-Smith, Islamicate Celestial Globes. Their History, Construction, and Use. Washington, D. C.: The Smithsonian Institution Press, 1985.
[35] Without diacritic points.
[36] See on this topic Toomer 1976, Rashed 1993, Rashed 1997a, and Rashed 1997b.
[37] This theoretical text on plane sundials sundials by Thābit ibn Qurra was edited and translated in Garbers 1936 and commented upon in Luckey 1937-38. It was also edited and translated in Morelon 1987, pp. 131-164.
[38] The textual tradition of the Arabic tradition of Euclid’s Optics is described in Kheirandish 1999.
[39] The extant manuscript copies of this text as listed by Hill (1991, pp. 178-179) are the following: Sülemaniye U. Kütüphanesi, Istanbul, Hagia Sophia 2755, item 1, Bodleian Library, Oxford 954 – Marsh 669, item 2, Leiden, Bibliotheek der Rijksuniversiteit Leiden, Codex Orientalis 499, ff. 74-87r, Gotha Universitäts-Bibliothek, Codex Gotha 1348, ff. 109-124r, and Florence Bibliotheca Medici Laurentiana, Codex Or. 152, ff. 82-90. MS Berlin 3306 must be added to this list, even though the pages corresponding to this reference are missing.
[40] See the French translation of this text in Mazahéri 1973.
[41] Al-Khzāzinī 1940, p. 4.
4.7 / 5. Votes 188
No votes so far! Be the first to rate this post.
Muslim Heritage:
Send us your e-mail address to be informed about our work.
This Website MuslimHeritage.com is owned by FSTC Ltd and managed by the Foundation for Science, Technology and Civilisation, UK (FSTCUK), a British charity number 1158509.
© Copyright FSTC Ltd 2002-2020. All Rights Reserved.
